Many websites/experts claim that the longer you hold your assets, the likelier your asset's return is closer to that predicted by the compound interest formula.
However, some other experts claim that this is wrong based on the Modern Portfolio Theory and in fact the probability the asset growth will be at least that will decrease as you hold your assets longer.
The chart below essentially shows how the probability distribution of asset growth changes over time with a portfolio of an expected return = 0.05 and risk = 0.2. In this simulation, the probability of you getting at least the expected amount as per compound interest is 46% on first year, 42% on fifth year, 38% on tenth year etc.
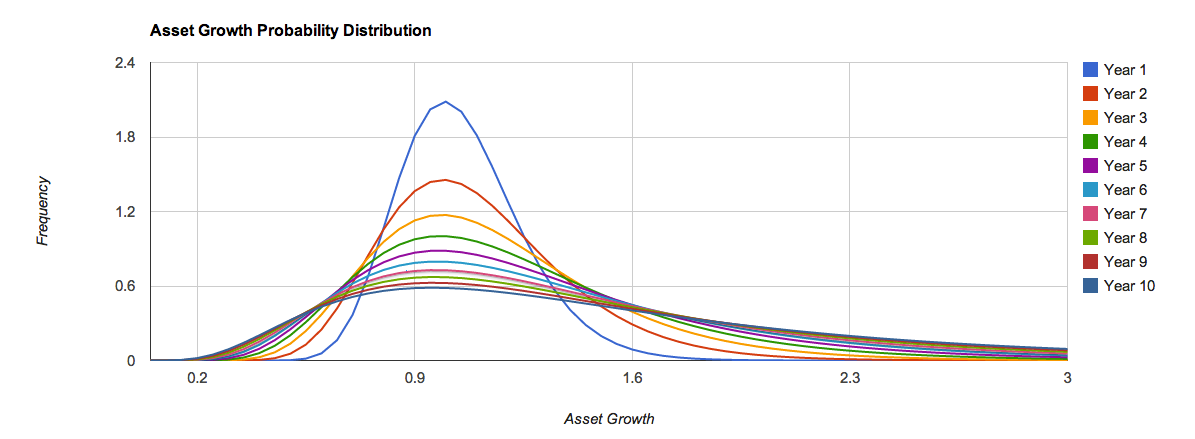
The simulation is available here
In fact, more disturbingly, the mode of asset growth (i.e. the most likely scenario) will be 100% on first year, 98% on fifth year and 95% on tenth year. I.e. the most likely scenario is that you lose money (this changes when the return/risk changes. For example, under return=0.05 and risk=0.1, the most likely scenario is that you gain).
My questions are:
a) Is this simulation a correct conclusion of Modern Portfolio Theory?
b) If it is correct, will using DCA change the behavior of asset growth? (To be more specific, will the most likely asset growth still be in the negative under a portfolio with e.g. return=0.05 and risk=0.2 when I use DCA?)

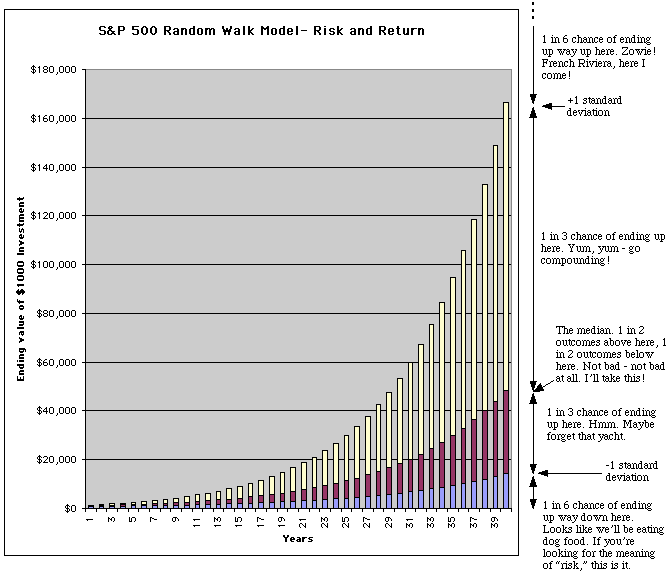
predicted by the compound interest formulawith the risk you assume. Secondly ask Warren Buffet. Thirdly your simulation has assumptions, which can go either way. So it boils down to how correct your assumptions are.