What is the Arbitrage opportunity and strategy here?

Question

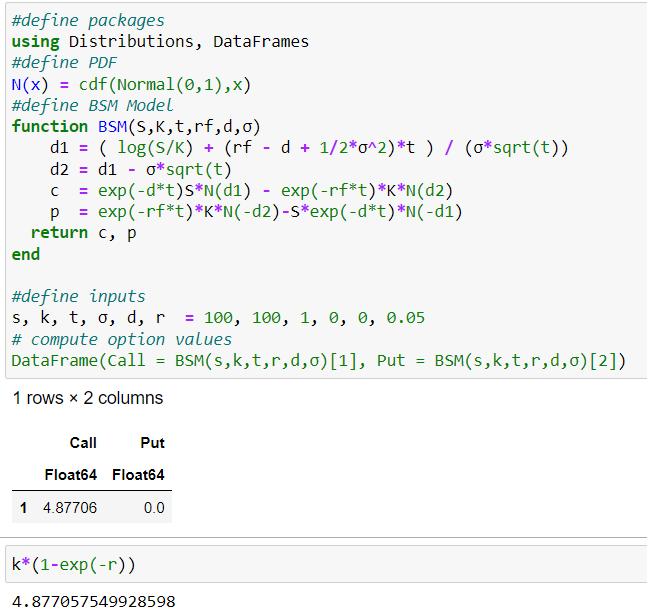
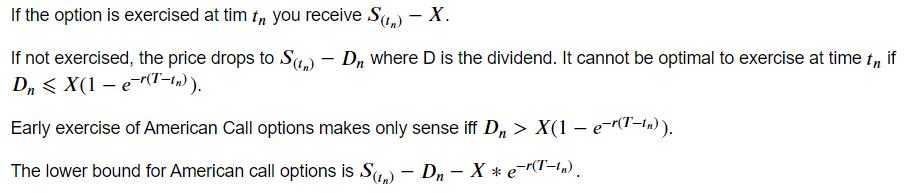1. Suppose that the current stock price is €100, the exercise price is €100, the annually compounded interest rate is 5 percent, the stock pays a €1 dividend in the next instant, and the quoted call price is €3.50 for a one year option. Identify the appropriate arbitrage opportunity and show the appropriate arbitrage strategy.

My attempted answer:

Early Exercise of American Calls on Dividend-Paying Stocks

When a company declares a dividend, it specifies that the dividend is payable to all stockholders as of a certain date, called the holder-of-record date. Two business days before the holder-of-record date is the ex-dividend date. To be the stockholder of record by the holder-of-record date, one must buy the stock by the ex-dividend date. The stock price tends to fall by the amount of the dividend on the ex-dividend date.

When a stock goes ex-dividend, the call price drops along with it. The amount by which the call price falls cannot be determined at this point in our understanding of option pricing. Since the call is a means of obtaining the stock, however, its price could never change by more than the stock price change. Thus, the call price will fall by no more than the dividend. An investor could avoid this loss in value by exercising the option immediately before the stock goes ex-dividend. This is the only time the call should be exercised early.

Another way to see that early exercise could occur is to recall that we stated that the lower bound of a European call on a dividendpaying stock is Max [itex][ 0, S'_0 - X(1 + 0.05)^-1][/itex] where $S'_0$ is the stock price minus the present value of dividends. X is the strike price $$100$. To keep things simple, assume only one dividend of the amount D, and that the stock will go ex-dividend in the next instant. Then $S'_0$ is approximately equal to $S_0 -D= $100-$1= $99 $ (since the present value of D is almost D). Since we would consider exercising only at-the-money call, assume that $S_0= $100 $ equals $X($100)$. Then it is easy to see that $S_0 - X= $100-$100 = 0$ could not exceed $S'_0 - X(1+0.05)^-1= $100 - $100(1+0.05)^-1= $3.76 $. By exercising the option, the call holder obtains the value $S_0 -X =$100 -$100= 0 $

Visit here for neat, legible
[Answer] [1]https://quant.stackexchange.com/q/74236/15605

Answer 1

You are confusing a lot of things here. The question (homework?) does not discuss early exercise of American options but is simply about arbitrage.

With regards to early exercise:

If the option is exercised at time t_n you receive S_(t_n)= - X. That would already be sufficient because in your example S = X = 100 and you will get 0. Getting zero for something that is not worthless (quoted price of 3.5) is suboptimal.

If not exercised, the price drops to S_(t_n) - D_n where D is the dividend. More generally, it is straightforward to show that it cannot be optimal to exercise at time t_n if D_n ⩽ X(1-e^{-r(T-t_n)}). It is not a coincidence that X(1-e(-0.05)) ≈ 4.88 which was your deleted Black Scholes pricing screenshot, because you had zero volatility and no dividends, in which case you do not need an option pricing model. This is also quick to verify with a bit of code (Julia).

#define packages
using Distributions, DataFrames
#define PDF
N(x) = cdf(Normal(0,1),x)
#define BSM Model 
function BSM(S,K,t,rf,d,σ)
    d1 = ( log(S/K) + (rf - d + 1/2*σ^2)*t ) / (σ*sqrt(t))
    d2 = d1 - σ*sqrt(t)
    c  = exp(-d*t)S*N(d1) - exp(-rf*t)*K*N(d2)
    p  = exp(-rf*t)*K*N(-d2)-S*exp(-d*t)*N(-d1) 
  return c, p
end

#define inputs
s, k, t, σ, d, r  = 100, 100, 1, 0, 0, 0.05
# compute option values
DataFrame(Call = BSM(s,k,t,r,d,σ)[1], Put = BSM(s,k,t,r,d,σ)[2])

Early exercise of American Call options makes only sense iff D_n > X(1-e(-r)).

Arbitrage

You do have, as the question suggests, arbitrage though. Since I assume it is homework, I will only hint the result.

The lower bound for American call options is S_(t_n)-D_n - X*exp^{-r(T-t_n)}.

However, 3.5 < 3.87, in which case the call option is less than the theoretical minimum. An arbitrageur can buy the call and short the stock, to get a cashflow equal to the proceeds of the stock minus the cost of the call. Invested at the prevailing one-year interest rate, you can get a certain payoff at the end of the year, where the option expires. If the stock price is above the strike price, the arbitrageur exercises the option, closes out the short position and makes a profit equal to the difference between sthe investment and strike.

If the stock is less than strike, the stock is bought in the market and the short position is closed out - this will yield an even greater profit.

Any introductory option pricing book like Hull explains this in more detail.

Apologies notation wise, but Money stackexchange does not support Latex.

A numerical example in code:

Answer 2

The idea that an option's price should trade for some hypothetical amount ($4.38) is erroneous because an option's price is determined by the market. If there is net buying, it increases. If there is net selling, it decreases. The current price is its value not some Black Scholes extrapolation.

Another way to look at this is that if an ITM American call has time premium remaining, it makes no sense to exercise it because doing so throws away that time premium. Just sell the call and buy the stock.

You can only arbitrage an ITM long call if you can buy it for less than its intrinsic value and that's a rare event for retail traders. Furthermore, that's discount arbitrage, not dividend arbitrage.