Why is the theta highest for the option at the money(22.50 in the screenshot), but decreases as we go further out of the money?
I understand that ITM have little time value, so they will have small time decay(theta), but why OTM has a lesser theta than ATM?
There's a key assumption made in the calculation of theta: that the future price movement of the underlying is a random walk.
The amount of life left in the option times the volatility of the underlying creates a probability distribution of the price of the underlying at expiration. At any given price point, you can calculate the theta of the option.
The at-the-money values are the most likely. The way-in-or-way-out-of-the-money values are much less likely.
Theta is constructed mathematically to decay linearly over time. So the strikes with the most theta lose the most theta each day.
If you are looking for a more intuitive answer, the OTM calls have less theta than the ATM calls because, while they are both 100% time value, the OTM calls cost much less. So it's 100% of a smaller number. Remember decay is linear.
If you look at the definition of time value on Wikipedia, you may notice this line: Time value "can be thought of as the price an investor is willing to pay for potential upside."
https://en.wikipedia.org/wiki/Option_time_value
You are right to think that the time value is increasing as the moneyness increases from deeply OTM to ATM. Once the moneyness crosses the strike price threshold, per wiki's interpretation, the upside potential decreases as the underlying price moves away from the strike price. Think it as if an option is already deep in the money, the chance of it getting further ITM is slim. Hence the willingness to pay for that chance (aka. the upside potential) decreases.
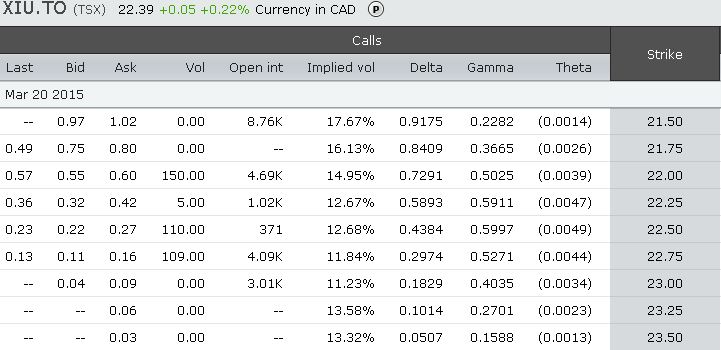
I understand that ITM have little time value, so they will have small time decay(theta), but why OTM has a lesser theta than ATM?
The Time value represents uncertainty. That uncertainty decreases the farther away from ATM you get (in either direction). At-the-money, there is roughly a 50% chance that the option expires worthless. As you get deeper in-the-money, the change that is expires worthless decreases, so there is less uncertainty (there is more certainty that the option will pay off). As you go deeper OTM, the probability that the option expires worthless increases, so there is also less uncertainty.
At the TTM decreases, the uncertainty (theta) decreases as well, since there is less time for the option to cross the strike from either direction. Similarly, as volatility decreases, theta decreases, since low-volatility stocks have a less change of crossing the strike.
