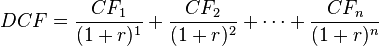0

Is there an exact formula for that if we can predict the growth accurately?

For example:

Using discounted cash flow analysis, the impact of earnings growth and inflation can be evaluated. Using constant historical earnings growth rate of 3.8 and post-war S&P 500 returns of 11% (including 4% inflation) as the discount rate, the fair P/E is obtained as 14.42. A stock growing at 10% for next five years would have a fair P/E of 18.65.

How come a 11% returns correspond to 14.42 P/E ratio? 100%/14.42 is not 11%

Improve this question
2

1 Answer 1

Reset to default
4

So, the price-earnings ratio is price over earnings, easy enough. But obviously earnings are not static. In the case of a growing company, the earnings will be higher in the future. There will be extra earnings, above and beyond what the stock has right now. You should consider the future earnings in your estimate of what the company is worth now.

One snag: Those extra earnings are future money. Future-money is an interesting thing, it's actually worth less than present-money- because of things like inflation, but also opportunity cost. So if you bought $100 in money that you'll have 20 years from now, you'd expect to pay less than $100. (The US government can sell you that money. It's called a Series EE Savings Bond and it would cost you $50. I think. Don't quote me on that, though, ask the Treasury.)

So you can't compare future money with present-money directly, and you can't just add those dollars to the earnings . You need to compute a discount. That's what discounted cash-flow analysis is about: figuring out the future cash flow, and then discounting the future figuring out what it's worth now.

The actual way you use the discount rate in your formula is a little scarier than simple division, though, because it involves discounting each year's earnings (in this case, someone has asserted a discount of 11% a year, and five years of earnings growth of 10%). Wikipedia gives us the formula for the value of the future cash flow:

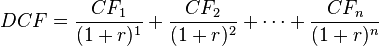
discounting formula here

essentially adding all the future cash flows together, and then discounting them by a (compounded) rate. Please forgive me for not filling this formula out; I'm here for theory, not math. :)

Improve this answer
2
  • The problem is if the company grows by 11% and pay no dividen, couldn't that complicated formula be simplified?
    – user4951
    Commented Jan 29, 2013 at 11:44
  • 1
    Paying a dividend is irrelevant to the P/E ratio: the company has the cash, you can ignore whether they distribute it immediately. Can you simplify the formula? Depends! Do you know enough calculus to think Σ(CF[n]/((1+r)^n)) is simpler? How about if we replace CF[n] with CF[0] * (1+g)^(n) (where g is the earnings growth rate)? Maybe the substituted version could be shuffled... but it obscures the fact that a company's profits aren't symbols and formulas! It's a series of real-world transactions. (Besides, I'm rusty on my calculus.)
    – user296
    Commented Jan 29, 2013 at 14:59

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .