Comparing option deltas of significantly different underlyings

Question

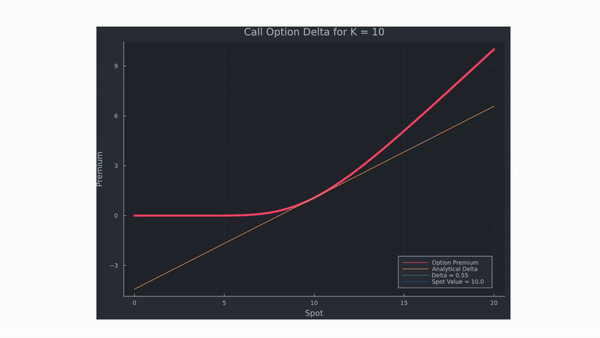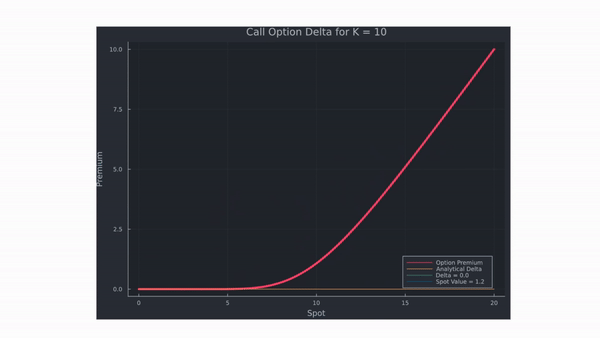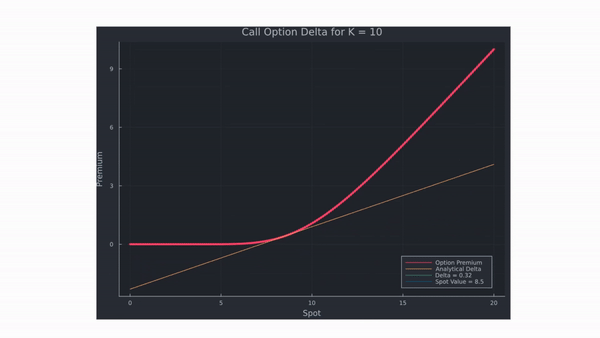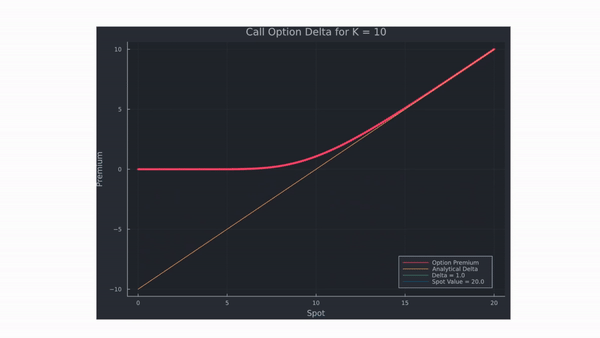
Please help me correct or confirm my intuition about options deltas. My understanding is that delta is the change in an option's price given a $1 change in the option's underlying. Moreover it's common to consider that the option's delta is a rough estimate of the probability that the option will be in the money at expiration. I struggle to understand the value of paying attention to delta when I consider the delta of options on two very different underlyings.

For example, as of this writing the delta for the May-19 TSLA 165 Put is -0.26. I take this to mean that this option's price will decrease by $0.26C should TSLA increase by $1. The delta for the May-05 6EM3 1.09 Put is -0.25 and similarly I take this to mean that this option's price will decrease by $0.25C should the 6EM3 currency future increase by $1. My struggle presents itself because while the TSLA equity moves many dollars in a day, the 6EM3 currency future moves fractions of cents in a day.

• Is my intuition off? If so, please help me correct it.
• How can we compare the deltas of options on different underlyings if delta is in terms of the $1 move of the underlying and different underlyings move very differently?
• Given that the 6EM3 currency future moves fractions of cents in a day, I would think that the likelihood of the option price moving $0.25C is very low since the underlying will almost certainly not move by $1. How do I reconcile this with the idea that delta represents probability?
• Is there an "improved" delta or completely other metric that captures something that can be more easily compared?
• Would normalizing underlyings make this simpler to intuit?

Answer 1

Assume two hypothetical underlyings, called A and B. Ignore for simplicity that Currency futures will be priced with Black 76, whereas TSLA would be priced with Black Scholes (the difference between the models is small anyways).

• A fluctuates widely most of the times, and at the money implied vol (ATM IV) is 80%.
• B is hardly moving and ATM IV is 5%.

If you price an option on both underlyings that is identical in terms (ATM, same expiry date - e.g. a year, both European call, same risk free rate and dividend) delta will be very different.

If rates and divs are 0, you will get a delta of just below 51 for the low vol underlying, and a delta of 65.54 for the high vol underlying. Therefore, if spot moves a lot, delta for an ATM option will be far from 50. You can find a more mathematical explanation and working computer code in this answer on quant SE.

In terms of (risk neutral) probability of ending up ITM, it is only a very rough proxy (see here for some details). Nonetheless, vol has a direct impact on that number.

You can see an interactive graphic (displayed below for convenience) showing and explaining in text what delta is mathematically here. The smaller the change in the underlying, the better the approximation delta offers.

To summarize, there is no need to "normalize" anything, and the value of delta accurately refers to the change in option price with respect to a small change in the underlying.

Answer 2

Without going into too much calculus, Delta is the derivative of the option price relative to the underlying price, so it does not require a $1 move and actually is more accurate with smaller moves. In fact the larger the move, the less accurate delta is (because it's non-linear).

Using delta to represent "probability" is tricky. It is true that options with low delta have a lower probability of expiry, but it is not mathematically equal.

How can we compare the deltas of options on different underlyings if delta is in terms of the $1 move of the underlying and different underlyings move very differently?

Delta is a relative measure, it does not necessarily require a $1 move. You would simply scale by the expected change in the underlying, e.g. by dividing it by 100 to get the expected move if the underlying moves $0.01, or multiplying by 10 if you expect the underlying to move by $10. Think of delta as the slope if you plotted the price of the option against the price of the underlying.

Given that the 6EM3 currency future moves fractions of cents in a day, I would think that the likelihood of the option price moving $0.25C is very low since the underlying will almost certainly not move by $1

Yes, so in this case, you might look at the expected move if the underlying moves by 0.001, which would be 0.00025 in this case.

If you're comfortable with the calculus, you can also look at gamma, which is the sensitivity of delta to the underlying price, and even use it to get a more accurate for moves of any size by using a 2nd order taylor series expansion.