The short of it is that bonds are valued based on a fundamental concept of finance called the "time value of money". Stated simply, $100 one year from now is not the same as $100 now. If you had $100 now, you could use it to make more money and have more than $100 in a year. Conversely, if you didn't invest it, the $100 would not buy as much in a year as it would now, and so it would lose real value. Therefore, for these two benefits to be worth the same, the money received a year from now must be more than $100, in the amount of what you could make with $100 if you had it now, or at least the rate of inflation. Or, the amount received now could be less than the amount recieved a year from now, such that if you invested this lesser amount you'd expect to have $100 in a year.
The simplest bonds simply pay their face value at maturity, and are sold for less than their face value, the difference being the cost to borrow the cash; "interest". These are called "zero-coupon bonds" and they're around, if maybe uncommon. The price people will pay for these bonds is their "present value", and the difference between the present value and face value determines a "yield"; a rate of return, similar to the interest rate on a CD.
Now, zero-coupon bonds are uncommon because they cost a lot. If I buy a zero-coupon bond, I'm basically tying up my money until maturity; I see nothing until the full bond is paid. As such, I would expect the bond issuer to sell me the bond at a rate that makes it worth my while to keep the money tied up. So basically, the bond issuer is paying me compound interest on the loan. The future value of an investment now at a given rate is given by FV = PV(1+r)t. To gain $1 million in new cash today, and pay a 5% yield over 10 years, a company or municipality would have to issue $1.629 million in bonds. You see the effects of the compounding there; the company is paying 5% a year on the principal each year, plus 5% of each 5% already accrued, adding up to an additional 12% of the principal owed as interest.
Instead, bond issuers can offer a "coupon bond". A coupon bond has a coupon rate, which is a percentage of the face value of the bond that is paid periodically (often annually, sometimes semi-annually or even quarterly). A coupon rate helps a company in two ways. First, the calculation is very straightforward; if you need a million dollars and are willing to pay 5% over 10 years, then that's exactly how you issue the bonds; $1million worth with a 5% coupon rate and a maturity date 10 years out. A $100 5% coupon bond with a 10-year maturity, if sold at face value, would cost only $150 over its lifetime, making the total cost of capital only 50% of the principal instead of 62%.
Now, that sounds like a bad deal; if the company's paying less, then you're getting less, right? Well yes, but you also get money sooner. Remember the fundamental principle here; money now is worth more than money later, because of what you can do with money between now and later. You do realize a lower overall yield from this investment, but you get returns from it quickly which you can turn around and reinvest to make more money. As such, you're usually willing to tolerate a lower rate of return, because of the faster turnaround and thus the higher present value.
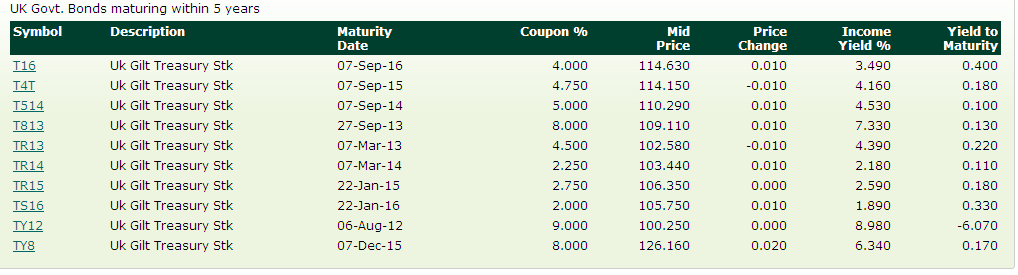
The "Income Yield %" from your table is also referred to as the "Flat Yield". It is a very crude measure, a simple function of the coupon rate, the current quote price and the face value (R/P * V). For the first bond in your list, the flat yield is (.04/114.63 * 100) = 3.4895%. This is a very simple measure that is roughly analogous to what you would expect to make on the bond if you held it for one year, collected the coupon payment, and then sold the bond for the same price; you'd earn one coupon payment at the end of that year and then recoup the principal. The actual present value calculation for a period of 1 year is PV = FV/(1+r), which rearranges to r = FV/PV - 1; plug in the values (present value 114.63, future value 118.63) and you get exactly the same result. This is crude and inaccurate because in one year, the bond will be a year closer to maturity and will return one less coupon payment; therefore at the same rate of return the present value of the remaining payout of the bond will only be $110.99 (which makes a lot of sense if you think about it; the bond will only pay out $112 if you bought it a year from now, so why would you pay $114 for it?).
Another measure, not seen in the list, is the "simple APY". Quite simply, it is the yield that will be realized from all cash flows from the bond (all coupon payments plus the face value of the bond), as if all those cash flows happened at maturity. This is calculated using the future value formula: FV = PV (1+r/n)nt, where FV is the future value (the sum of the face value and all coupon payments to be made before maturity), PV is present value (the current purchase price), r is the annual rate (which we're solving for), n is the number of times interest accrues and/or is paid (for an annual coupon that's 1), and t is the number of years to maturity. For the first bond in the list, the simple APY is 0.2974%. This is the effective compound interest rate you would realize if you bought the bond and then took all the returns and stuffed them in a mattress until maturity. Since nobody does this with investment returns, it's not very useful, but it can be used to compare the yield on a zero-coupon bond to the yield on a coupon bond if you treated both the same way, or to compare a coupon bond to a CD or other compound-interest-bearing account that you planned to buy into and not touch for its lifetime.
The Yield to Maturity, which IS seen, is the true yield percentage of the bond in time-valued terms, assuming you buy the bond now, hold it to maturity and all coupon payments are made on time and reinvested at a similar yield. This calculation is based on the simple APY, but takes into account the fact that most of the coupon payments will be made prior to maturity; the present value of these will be higher because they happen sooner. The YTM is calculated by summing the present values of all payments based on when they'll occur; so, you'll get one $4 payment a year from now, then another $4 in two years, then $4 in 3 years, and $104 at maturity. The present value of each of those payments is calculated by flipping around the future value formula: PV = FV/(1+r)t. The present value of the entire bond (its current price) is the sum of the present value of each payment: 114.63 = 4/(1+r) + 4/(1+r)2 + 4/(1+r)3 + 104/(1+r)4. You now have to solve for r, which is difficult to isolate; the easiest way to find the rate with a computer is to "goal seek" (intelligently guess and check).
Based on the formula above, I calculated a YTM of .314% for the first bond if you bought on Sept 7, 2012 (and thus missed the upcoming coupon payment). Buying today, you'd also be entitled to about 5 weeks' worth of the coupon payment that is due on Sept 07 2012, which is close enough to the present day that the discounted value is a rounding error, putting the YTM of the bond right at .40%. This is the rate of return you'll get off of your investment if you are able to take all the returns from it, when you receive them, and reinvest them at a similar rate (similar to having a savings account at that rate, or being able to buy fractional shares of a mutual fund giving you that rate).