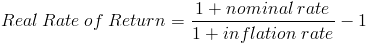Suppose i invest 100000 today.and suppose rate of interest is 12% and inflation rate is 6% . so what amount i will get after 2 year at the rate of 12% and inflation of 6%.
In other word you can say,how the rate of interest affects inflation rate.
Please help me to get this.I'm struggling for this for a long while. Any help will be appreciated. Thanks in advance.
Inflation doesn’t affect your nominal investment return at all, only its spending power. If you invest $100,000 for two years at 12% per year, you will have $125,440, whatever the inflation rate is. If the inflation rate for the things you want to buy (which may be different from the headline inflation rate) was 6% per year, then that $125,440 will only buy you as much as $111,641 would have at the time you made the initial investment.
10^5 * 1.06^2 = 112,360 most certainly is mathematically correct as to what 10^5 is after two years of inflation. 111,641 is the answer to a different problem: 1.12^2 / 1.06^2 = 1.11641153.
t + 2 to equal 100,000 today. Yes the math is correct isolated - but that is the answer to a different problem.
10^5 * 1.06^2 (which is 112,360) and 10^5 * 1.12^2/1.06^2 (which is 111,641).
With principal, interest rate and inflation
p = 100000
r = 0.12
i = 0.06
after two years you have
p (1 + r)^2 = 125440.00
However, accounting for inflation, in today's value that is
125440/(1 + i)^2 = 111641.15
This is the same as adjusting for inflation after each compounding period, which would be necessary if there were intervening cash flows.
year1 = p (1 + r)/(1 + i) = 105660.38
year2 = year1 (1 + r)/(1 + i) = 111641.15
See http://financeformulas.net/Real_Rate_of_Return.html
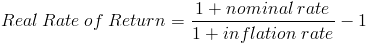
also https://money.stackexchange.com/a/56847/11768
The quick and dirty method you may find mentioned elsewhere is
p (1 + (r - i))^2 = 112360.00
but that's just lazy and wrong.
It is more rigorous to use an extra step calculating x
x = i (1 + r)/(1 + i)
p (1 + (r - x))^2 = 111641.15
Additional note by RonJohn
The Q&D method is wrong because (1 + (r - i))^2 where r=12% and i=6% reduces to 1.06^2, whereas 1.12^A grows faster than 1.06^A.
The balance of your investment investment account will grow at 12% APR no matter what the rate of inflation.
This means that after two years the account value will be 1.122 = 1.2544 times more than the starting balance.
But... since the value of money has -- in some economist's delusionally uniform world -- decreased by 6%/annum for two years, you now require 1.062 = 1.1236 times more than the starting balance just to be able to buy what you did two years ago.
You'd think that because 12% is 2x as large as 6% that you'd have 2x as much money every year. Not so, because compounding is a power function while ratios are linear.
The formula for the yearly percentage difference between a 12% investment and 6% inflation is calculated by ((1.12^A)-1)/((1.06^A)-1) - 2 where A is the number of years since the start.
This is derived from:
1.12^A/1.06^A
Correct: I've not mentioned your 100000 starting amount, because it's irrelevant. Multiply your starting balance (whatever it's size, whether 20 or 80 or 10^5 or 4.2*10^72) by 1.06^A and 1.12^A to get the values for each year A. And naturally, the .12 and .06 can be changed to any number you'd like depending on the investment and inflation rates.
