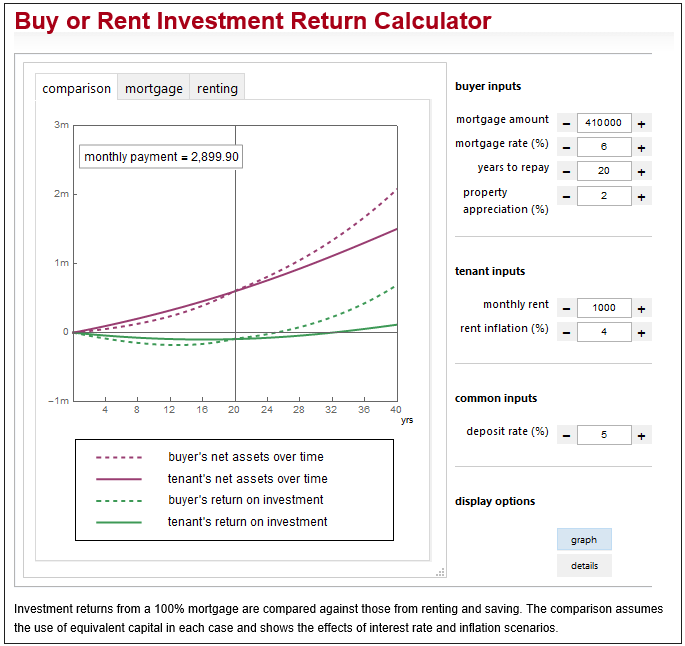
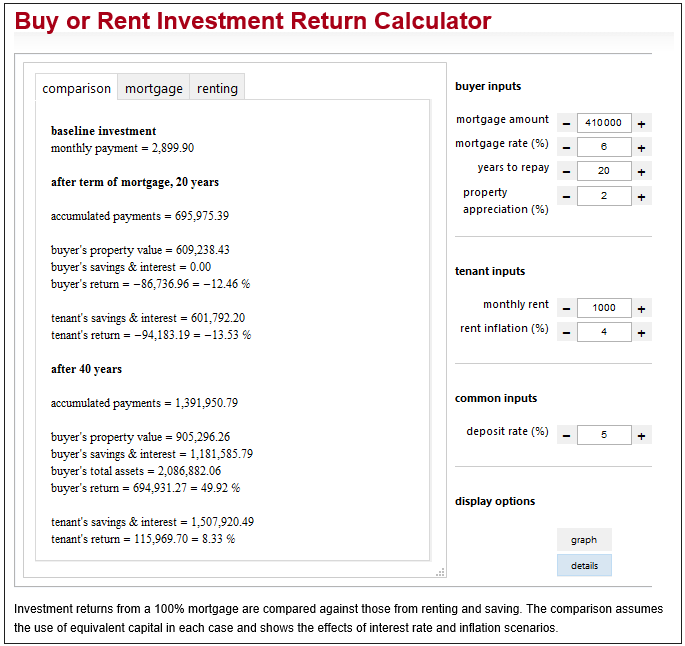
In most cases of purchases the general advice is to save the money and then make the purchase. Paying cash for a car is recommended over paying credit for example. For a house, getting a mortgage is recommended.
Is this because a house is so expensive, saving is not practical? Is it because the house is expected to appreciate in value? Then isn't a mortgage really just an option to own a property that you buy with borrowed money and you're betting that the house's price will increase?
In most cases of purchases the general advice is to save the money and then make the purchase. Paying cash for a car is recommended over paying credit for example. For a house, getting a mortgage is recommended.
Says who? These rules of thumb hide the actual equations behind them; they should be understood as heuristics, not as the word of god.
The Basics
The basic idea is, if you pay for something upfront, you pay some fixed cost, call it X, where as with a loan you need to pay interest payments on X, say %I, as well as at least fixed payments P at timeframe T, resulting in some long term payment IX.
Your Assumption
To some, this obviously means upfront payments are better than interest payments, as by the time the loan is paid off, you will have paid more than X. This is a good rule of thumb (like Newtonian's equations) at low X, high %I, and moderate T, because all of that serves to make the end result IX > X.
Counter Examples
Are there circumstances where the opposite is true? Here's a simple but contrived one: you don't pay the full timeframe. Suppose you die, declare bankruptcy, move to another country, or any other event that reduces T in such a way that XI is less than X. This actually is a big concern for older debtors or those who contract terminal illnesses, as you can't squeeze those payments out of the dead. This is basically manipulating the whole concept.
Let's try a less contrived example: suppose you can get a return higher than %I. I can currently get a loan at around %3 due to good credit, but index funds in the long run tend to pay %4-%5. Taking a loan and investing it may pay off, and would be better than waiting to have the money, even in some less than ideal markets. This is basically manipulating T to deal with IX.
Even less contrived and very real world, suppose you know your cash flow will increase soon; a promotion, an inheritance, a good market return. It may be better to take the loan now, enjoy whatever product you get until that cash flows in, then pay it all off at once; the enjoyment of the product will make the slight additional interest worth it. This isn't so much manipulating any part of the equation, it's just you have different goals than the loan.
Home Loan Analysis
For long term mortgages, X is high, usually higher than a few years pay; it would be a large burden to save that money for most people.
%I is also typically fairly low; P is directly related to %I, and the bank can't afford to raise payments too much, or people will rent instead, meaning P needs to be affordable. This does not apply in very expensive areas, which is why cities are often mostly renters.
T is also extremely long; usually mortgages are for 15 or 30 years, though 10 year options are available. Even with these shorter terms, it's basically the longest term loan a human will ever take. This long term means there is plenty of time for the market to have a fluctuation and raise the investments current price above the remainder of the loan and interest accrued, allowing you to sell at a profit.
As well, consider the opportunity cost; while saving money for a home, you still need a place to live. This additional cost is comparable to mortgage payments, meaning X has a hidden constant; the cost of renting. Often X + R > IX, making taking a loan a better choice than saving up.
Conclusion
"The general advice" is a good heuristic for most common human payments; we have relatively long life spans compared to most common payments, and the opportunity cost of not having most goods is relatively low.
However, certain things have a high opportunity cost; if you can't talk to HR, you can't apply for jobs (phone), if you can't get to work, you can't eat (car), and if you have no where to live, it's hard to keep a job (house). For things with high opportunity costs, the interest payments are more than worth it.
First, who is saying that it is a better option? In general it is best to pay cash for things when you can. I think the reality is that for most people owning a house would be very difficult without some sort of financing.
That said, one argument for financing a house these days even if you could afford to pay cash is that the interest rates are very low. For a 30-year fixed loan you can borrow money under 4.5% APR with decent credit. If you are willing to accept even a little risk you could almost certainly invest that same money and get a return higher than 4.5%. With the US mortgage interest tax deduction the numbers are even more favorable for financing.
Those rates look even more attractive when you consider you are paying for the house with today's dollars and paying back the loan with dollars from up to 30 years in the future, which will be worth much less.
First, as others have commented, the idea that getting a mortgage to buy a house is always a good idea is false. It depends on a number of factors including the current interest rate, what you think the future interest rate will do over the life of your mortgage, the relative cost of renting vs. buying, and how long you would stay in the house that you bought.
To the extent that a mortgage for a house is more often recommended than buying other goods on credit, it is for these reasons:
Except for #1 above, you could and can find other situations where taking a loan makes more sense than buying in cash. This more true if you have the resources and the skill to invest money at a rate that beats the interest rate you pay to the creditor. The general advice not to try this rests in the fact that most people don't have the resources or the skill to actually make this pay off, especially on high-interest rate loans or over short time periods.
You can explore the scenarios in which it is better to rent or to buy using this application:
http://demonstrations.wolfram.com/BuyOrRentInvestmentReturnCalculator/
In the possibly unlikely scenario shown below, at the term of the mortgage (20 years) the tenant and the buyer have practically the same return on investment. At this point the tenant's savings would be sufficient to buy a house equivalent to the buyer's, and this would be the advisable course of action (based on the figures alone).
The benefit of saving first to buy a thing (anything) is that it costs you less - you aren't paying interest; depending on the economic climate (interest rate vs inflation) and the cost of the thing, deferring a purchase may allow you to afford something better when you can eventually buy it without borrowing.
The benefit of borrowing to buy a thing is that you get to enjoy it right away.
For many purchases like electronics, household appliances, and so forth, it shouldn't take all that long to save enough to pay in cash, so you're not significantly deferring the benefit of having it.
Cars, being more expensive, may take longer to save, and you may not be prepared (or able) to wait until you can pay cash for one, so borrowing allows you to gain the benefits of having one sooner, but it comes at a cost. If you can afford the financing cost, then it can make sense to borrow for a car, but you're always financially better off to save first and then buy.
Houses are generally the most expensive thing a person will buy, and for most people, it will require an impractically long time to save up enough to buy in cash. Since lenders generally view mortgages as low-risk investments, mortgages are typically the least expensive money you can get (lowest interest rates). This is what makes borrowing to buy a house a sensible option (assuming you can keep up the mortgage and all other associated expenses). Also, most properties appreciate in value over time; once you've bought in, you acquire the potential to benefit from appreciation when it comes time to sell (it's never guaranteed, but it is fairly certain in most cases); just about anything else you might buy will depreciate from the moment you buy it. Deferring a home purchase can make an eventual purchase more difficult (depending on market) because prices sometimes rise faster than average inflation.
It's been said that it's better to buy than rent, and the best time to buy was last year. These bits of wisdom are true in many cases, and used to favor the argument to borrow and buy now, but you always have to analyze the specifics of each individual case. What is your employment situation and income prospect over the next 5, 10, 20 years? What does the rental market look like in your area? How much will your desired house cost? How much have you saved so far toward a down payment? What will your cash flow look like after you buy (mortgage, taxes, utilities, maintenance, food, clothing, car and/or other transportation for yourself and any dependents, travel and entertainment)? Will you still have something left over for retirement savings, "rainy day" savings, discretionary spending? In general the question is not so much whether or not to borrow to buy a house, but whether or not to buy vs rent, and when to buy.
