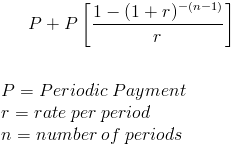If I were to win the lottery and the present winnings were less than twenty million paid out over a time period of twenty years, how would I theoretically find the present value amount?
Add a comment
|
2 Answers
If you are using an Excel, the Function PV should be able to easily calculate this.
Excel Formulae PV = (Rate,Nper,Pmt,Fv,Type)
Where
Rate: Rate of return. In this case you can use Inflation or assumed rate that would cost you. Say 3-5%. Note the Rate has to be for Nper. i.e. in Nper if you are counting yearly payments, then rate is yearly, if you are counting as monthly, then the rate should be monthly.NPer: Number of periods. If yearly in your case it would be 20. If Monthly 20*12, if Quarterly 20*4 etc.
Pmt: Expected Payments for Nper. If you are saying 20 million over 20 years, it would be 1 million per year.
Fv and Type can be blank
So assuming a rate of 3%, and yearly payments of 1 million over 20 years.
PV = $14,877,474.86 [It would show negative, just ignore the sign]
-
You have calculated the present value for an ordinary annuity, so effectively you have to wait a year before receiving the first payment. That's more like how a re-payment would work, (paying back a loan). Commented Feb 25, 2016 at 16:43
-
Adding details. With
p = 1000000,r = 0.03andn = 20present value for an ordinary annuity isp ((1 - (1 + r)^-n)/r) = 14,877,474.86. By contrast, for an 'annuity due' the present value isp + p ((1 - (1 + r)^-(n - 1))/r) = 15,323,799.11Commented Feb 25, 2016 at 16:48
The example from the following website: Investopedia -
Calculating The Present And Future Value Of Annuities
specifically the section 'Calculating the Present Value of an Annuity Due' shows how the calculation is made. Using their figures, if five payments of $1000 are made over five years and depreciation (inflation) is 5%, the present value is $4545.95
There is also a formula for this summation, (ref. finance formulas)
p = 1000
r = 0.05
n = 5
p + p ((1 - (1 + r)^-(n - 1))/r) = 4545.95
answered Feb 25, 2016 at 16:34
