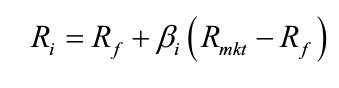0

Let say I want to invest 1000€ in cryptocurrencies in order to get 2000€

In the famous CAPM, the relationship between risk and rates of returns in a security is described as follows:

R_i = R_f+\Beta_i(R_{mkt}-R_f

For a security i , its returns is defined as R i and its beta as β i . The CAPM defines the return of the security as the sum of the risk-free rate R f and the multiplication of its beta with the risk premium. The risk premium can be thought of as the market portfolio's excess returns exclusive of the risk-free rate.

Beta is a measure of the systematic risk of a stock; a risk that cannot be diversified away. In essence, it describes the sensitivity of stock returns with respect to movements in the market. For example, a stock with a beta of zero produces no excess returns regardless of the direction the market moves—it can only grow at the risk-free rate. A stock with a beta of 1 indicates that the stock moves perfectly with the market.

My question is : can we have a Beta greater than 1 ? Would it mean that the stock moves "more than perfectly" with the market ?

If I have no risk free assets, would the formula to have 2000€ back be :

enter image description here

What sense would it have ?

Improve this question

1 Answer 1

Reset to default
1

For starters, the risk-free rate has nothing to do with stocks. It would be independent of anything. It pays out the same return in all states of nature. The definition of a risk-free asset is that regardless of how the universe turns out, including a meteor striking the Earth killing everyone but the recipient, then the payout would happen exactly as planned. One could imagine a computer still being on, connected to a power supply and printing a check. Most people use the 90-day t-bill as the risk-free rate. A beta greater than one implies it is more volatile than the market, not that it moves more perfectly.

The CAPM should not be used for this. Cryptocurrencies should not be used with this model because they have valuation dynamics related to the new issue of coins. In other words, they have non-market price movements as well as market price movements.

In general, you should not use the CAPM because it doesn't work empirically. It is famous, but it is also wrong. A scientific hypothesis that is not supported by the data is a bad idea. My strong recommendation is that you read "The Intelligent Investor," by Benjamin Graham. It was last published in 1972, and it is still being printed. I believe Warren Buffett wrote the current forward for it. Always go where the data supports you and never anywhere else, no matter how elegant.

Finally, unless you are doing this like a trip to Vegas, for fun and willing to take the losses, I would avoid cryptocurrencies because you don't know what you are doing yet. It is obvious from the posting. I have multiple decades working in every type of financial institution and at every level, bottom to top. I also have a doctorate, and I am an incredible researcher. I am professionally qualified in three different disciplines.

If you want to learn how to do this, start with the "Intelligent Investor." Get a basic book on accounting and learn basic accounting. Pick up economics textbooks at least through "Intermediate" for both microeconomics and macroeconomics. Get William Bolstad's book "Introduction to Bayesian Statistics." You will need them for reasons that go very far beyond this post. Trust me; you want to master that book. Find a statistician and ask them to teach it to you as a special topics course. It will help you as both either a Marine officer or a Naval officer. Then after that pick up a copy of "Security Analysis." Either the 1943 copy (yes it is in print) by Benjamin Graham if you feel good about accounting, or the 1987 copy by Cottle under the Graham/Dodd imprimatur. Then, if you are still interested in cryptocurrencies and they will be blasé by then, then pick up an economics textbook on money. If I were you, I would learn about Yap money, commodity money, and prison money first, then you might understand why a cryptocurrency may not be an investment for you.

Improve this answer
8
  • "In general, you should not use the CAPM because it doesn't work empirically. It is famous, but it is also wrong." Well, I wouldn't go that far. There is evidence both supporting it an denouncing it. I am a Graham investor myself, and even blindly applying Graham's rules can lead you down the wrong path (e.g. value traps). There are flaws in all methodologies if you look hard enough.
    – tendim
    Commented May 30, 2017 at 14:43
  • Actually, I have a paper out on a population test of the theory. It is -mathematically and empirically unsupportable. I also show there is a very subtle math error in its derivation. I tested every trade in CRSP from 1925-2013 minus things like shell companies.
    – Dave Harris
    Commented May 30, 2017 at 14:47
  • @tendim The empirical test, which follows from Mandelbrot's 63 paper and Markowitz's later paper papers.ssrn.com/sol3/papers.cfm?abstract_id=2653151. Ironically Markowitz himself found this but didn't realize that when you take the log approximation you change the distribution. It excludes a variance as existing.
    – Dave Harris
    Commented May 30, 2017 at 14:49
  • @tendim In this paper, papers.ssrn.com/sol3/papers.cfm?abstract_id=2828744 , I derive all return distributions. I am about to revise and submit for publication. I also replaced Black-Scholes and empirically tested the replacement. It works, Black-Scholes does not.
    – Dave Harris
    Commented May 30, 2017 at 14:50
  • @tendim Find ONE paper in the literature that accepts the hypothesis that the CAPM is valid. Look really hard. Then look at Fama-MacBeth and Fama-French and you will see there has never been a validation study. It is the same reason the APT never worked. Mandelbrot was correct in 1963, there is no mean return. I just showed it is impossible, except for single period discount bonds and bonds where the cash flows are consumed.
    – Dave Harris
    Commented May 30, 2017 at 14:53

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .