Let's assume I hold a bond that yields 3% for some time. Based on my calculations $100 are working in the investment. So it makes me $3 each year.
Now let's assume the yields have been increased so I can buy bonds that yield 4%. The bond I currently own can be sold for $80.
Should I sell my bond for $80 to buy the 4% bond so my investment would make $3.2 each year instead of $3?
Or consider the opposite: yields fell to 2% and my bond can be sold for $160. If I sell my 3% bond for $160 and buy the 2% one, my money would earn $3.2 again instead of $3. Should I do it?
My general goal is to maximize the income I can make with my money. Is maximizing income a good idea at all?
(Let's ignore the default risk of the bonds for a moment)
EDIT1:
I don't think fully understand the answers so far.
But here is my thought chain detailed:
You mentioned NPV, but I thought it's just a name of a function you need to turn zero, when solving for the IRR. Bonds with fixed coupons have a fixed internal return rate (IRR) since all future cash flows are proporitonal to the initial investment. IRR is the interest rate of a hypotetical savings account you would deposit your investments to and withdraw some from as the coupons mature. So you invest, it earns interest then you withdraw some, the remaining earn interest and so on... At the maturity date you'll exactly have the amount of the last payment.
I did a spreadsheet showing my calculations for a hypothetical case:
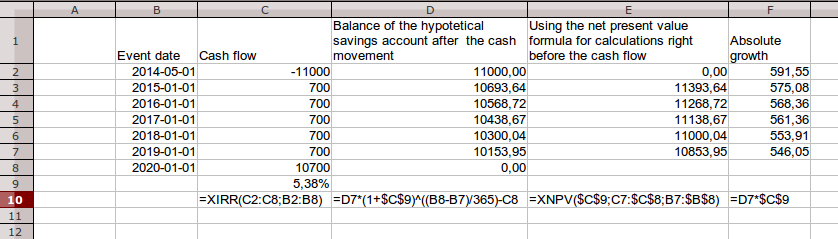
It turned out that XNPV function gives the very same values except that it calculates using future payments while I calculated the present value based on past cash movements with negative sign.
Should the future payments change, so the yields will, and the value on both columns will adjust accordingly as well.
So basically I calculated NPV already, just didn't know...
What I ask about is the last column. For any given moment of time I can tell the NPV and the rate it's making money.
Based on the IRR of the new bond's cash flows, and the selling price of a particular investment I can predict the new growth rate. If it provides higher growth I would switch.
Indeed if my investments' NPV is $100 and if I sell it for $80 I indeed lost $20. But my question is about maximizing NPV*yield product and not the absolute wealth. (let's ignore the default risk for a moment)
WRT my financial situation I'm a worker who earns his money, and I invest the surplus. The final goal is building a retirement portfolio that provides high income.
EDIT2: I've found that maximizing the growth rate isn't necessarily a good idea.

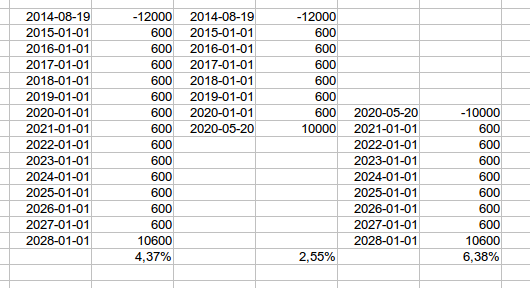
government bondsmeans US. It is considered risk free, but it isn't but the chances of US defaulting is less. So are many other countries. But yield and bond price move in tandem, so taking a real scenario is much helpful then assuming a hypothetical scenario.