Does it make sense to trade my GOOGL shares for GOOG and pocket the difference?

Question

I owned some GOOG shares at the split so now I have GOOG and GOOGL. Does it make sense to sell all my GOOGL shares, and buy GOOG shares and pocket the difference; presuming that the difference is greater than the transaction costs? Then get reimbursed by Google AGAIN for the difference after a year? Theoretically, I could trade the spread throughout the year, right?

Answer 1

How does it work*?

To keep it simple, let's say that A shares trade at 500 on average between April 2nd 2014 and April 1st 2015 (one year anniversary), then if C shares trade on average:

• above 495 (less than 1% discount), you'll get nothing
• between 490 and 495 (1-2%), you'll get 20% of the difference (between 500 and 49x)
• between 485 and 490 (2-3%), you'll get 40% of the difference
• between 480 and 485 (3-4%), you'll get 60% of the difference
• between 475 and 480 (4-5%), you'll get 80% of the difference
• below 475 (more than 5% discount), you'll get $25

The payment will be made either in cash or in shares within 90 days.

Can you make money out of it?

1. It may work: let's say you manage to buy the Cs at a 2% discount to As now, say 490 vs 500. At the end of the period, if Cs have traded on average at a 0.8% discount to As, you don't get anything, so the result of your trade will depend on whether Cs are trading at less than 2% discount vs. As when you unwind your trade. However, if the average discount has been 0.8%, then you would have had opportunities to exit at a gain, but that would require your monitoring the spread carefully.
2. It may not work: you buy at a 2% discount but the market values the voting rights at more than 5% per share (it can happen - see for example Discovery Communications C shares trading at a discount of 6.1% vs A shares as I write this) - let's say 8% per share on average over the year: you get paid 5% but have lost 6% which results in a net loss of 1% for the trade.

The difficulties come from the fact that the formula is based on an average price over a year, which is not directly tradable, and that the spread is only covered between 1% and 5%.

In practice, it is unlikely that the market will attribute a large premium to voting shares considering that Page&Brin keep the majority and any discount of Cs vs As above 2-3% (to include cost of trading + borrowing) will probably trigger some arbitrage which will prevent it to extend too much. But there is no guarantee.

How is it going so far?

FYI here is what the spread has looked like since April 3rd:

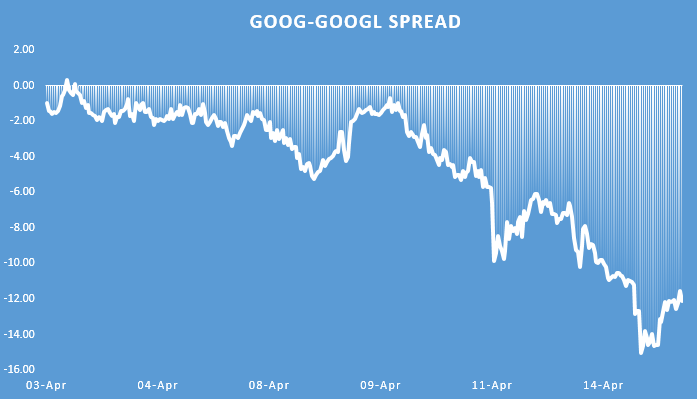
_{* details in the section called "Class C Settlement Agreement" in the S-3 filing}

Answer 2

It appears very possible that Google will not have to pay any class C holders the settlement amount, given the structure of the settlement. This is precisely because of the arbitrage opportunity you've highlighted. This idea was mentioned last summer in Dealbreaker.

As explained in a Dealbook article:

The settlement requires Google to pay the following amounts if, one year from the issuance of the Class C shares, the value diverges according to the following formula:

• If the C share price is equal to or more than 1 percent, but less than 2 percent, below the A share price, 20 percent of the difference;

• If the C share price is equal to or more than 2 percent, but less than 3 percent, below the A share price, 40 percent of the difference;

• If the C share price is equal to or more than 3 percent, but less than 4 percent, below the A share price, 60 percent of the difference;

• If the C share price is equal to or more than 4 percent, but less than 5 percent, below the A share price, 80 percent of the difference.”

• If the C share price is equal to or more than 5 percent below the A share price, 100 percent of the difference, up to 5 percent.

...

If the Class A shares trade around $450 (after the split/C issuance) and the C shares trade at a 4.5 percent discount during the year (or $429.75 per share), then investors expect a payment of: 80 percent times $450 times 4.5 percent = $16.20. The value of C shares would then be $445.95 ($429.75 plus $16.20). But if this is the new trading value during the year, that’s only a discount of less than 1 percent to the A shares. So no payment would be made. But if no payment is made, we are back to the full discount and this continues ad infinitum.

In other words, the value of a stock can be displayed as:

{equity value} + {dividend value} + {voting value} + {settlement value} = {total share value}

If we ignore dividend and voting values, and ignore premiums and discounts for risk and so forth, then the value of a share is basic equity value plus anticipated settlement payoff. The Google Class C settlement is structured to reduce the payoff as the value converges. And the practice of arbitrage guarantees (if you buy into at least semi-strong EMH) that the price of C shares will be shored up by arbitrageurs that want the payoff.

The voting value of GOOGL is effectively zero, since the non-traded Class B shares control all company decisions. So the value of the Class A GOOGL voting is virtually zero for the time being. The only divergence between GOOGL and GOOG price is dividends (which I believe is supposed to be the same) and the settlement payoff. Somebody who places zero value on the vote and who expects dividend difference to be zero should always prefer to buy GOOG to GOOGL until the price is equal, disregarding the settlement.

So technically someone is better off owning GOOG, if dividends are the same and market prices are equal, just because the vote is worthless and the nonzero chance of a future settlement payoff is gravy.

The arbitrage itself is present because a share that costs (as in the article) $429.75 is worth $445.95 if the settlement pays out at that rate. The stable equilibrium is probably either just before or just after the threshold where the settlement pays off, depending on how reliably arbitrageurs can predict the movement of GOOG and GOOGL.

If I can buy a given stock for X but know that it's worth X+1, then I'm willing to pay up to X+1. In the google case, the GOOG stock is worth X+S, where S is an uncertain settlement payment that could be zero or could be substantial. We have six tiers of S (counting zero payoff), so that the price is likely to follow a pattern from X to X+S5 to X-S5+S4 to X-S4+S3, and climbing the tier ladder until it lands in the frontier between X+S1 and X+S0. Every time it jumps into X+S1, people should be willing to pay that new amount for GOOG, so the price moves out of payoff range and into X+S0, where people will only pay X.

I'm actually simplifying here, since technically this is all based on future expectations. So the actual price you'd pay is expressed thus:

{resale value of GOOG before settlement payoff = X} +

( {expectation that settlement payoff will pay 100% of difference = S5} * {expected nominal difference between GOOG and GOOGL = D} ) +

({S4} * {80% D}) +

({S3} * {60% D}) +

({S2} * {40% D}) +

({S1} * {20% D}) +

({S0} * {0% D})

= {price willing to pay for Class C GOOG = P}

Plus you'd technically have to present value the whole thing for the time horizon, since the payoff is in a year. Note that I've shunted any voting/dividend analysis into X. It's reasonable to thing that S5, S4, S3, and maybe S2 are nearly zero, given the open arbitrage opportunity. And we know that S0 times 0% of D is zero. So the real analysis, again ignoring PV, is thus:

P = X + (S1*D)

Which is a long way of saying: what are the odds that GOOG will happen to be worth no more than 99% of GOOGL on the payoff determination date?

Answer 3

Too much fiddling with your portfolio if the difference is 3-4% or less (as it's become in recent months). Hands off is the better advice. As for buying shares, go for whichever is the cheapest (i.e. Goog rather than Googl) because the voting right with the latter is merely symbolic. And who attends shareholders' meetings, for Pete's sake?

On the other hand, if your holdings in the company are way up in the triple (maybe even quadruple) figures, then it might make sense to do the math and take the time to squeeze an extra percentage point or two out of your Googl purchases. The idle rich occupying the exclusive club that includes only the top 1% of the population needs to have somethinng to do with its time. Meanwhile, the rest of us are scrambling to make a living--leaving only enough time to visit our portfolios as often as Buffett advises (about twice a year).