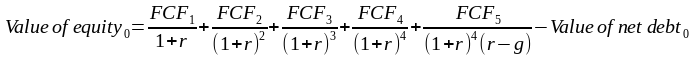Discounted cash flow: meaning of terminal value formula

Question

I am reading a book about stock valuation using fundamental analysis — Accounting for Value by Stephen Penman. The following discounted cash flow (DCF) formula appears in chapter 2 with almost no explanation:

Where r is the required rate of return, and g is the perpetual growth rate.

I am mostly confused about the "terminal value" — the fifth term: FCF_5 / [(1 + r)^4 (r - g)]. From my understanding, it is meant to represent all future discounted free cash flows from t=5 to t=∞. I understand that the division by (r - g) is for calculating a growing perpetuity. What I do not understand is the FCF_5 / (1 + r)^4 part. Why is there a division by (1 + r)^4? Why is it not, say, a division by (1 + r)^5 instead?

Answer 1

Each term in the equation is an end of year cash flow discounted back to the present using some factor. Take cash flows realized at the end of year 1, discount those back to the present at 1+r. Take cash flows realized at the end of year 2, discount those back to the present at a 2 year compounded discount rate (1 + r)^2...so on and so forth.

The denominator of the last term breaks the convention by saying ok, instead of starting at the end of the fifth year and discounting cash flows using the standard compounded rate through 5 years, let's start at the beginning of year 5, take the compounded rate through four years and adjust it by (r-g) to represented discounted growth in perpetuity. If you adjusted the perpetuity rate by (1 + r)^5 instead of (1 + r)^4 you'd be double discounting.

Answer 2

A simpler way to think about this is to break the terms apart a bit, and first think of the terminal value as if it were to be calculated for this year, instead of year 5.

Assume a single-year cashflow is $100, and that $100 is expected to grow annually by 2%. Annual required rate is, say, 4%.

The terminal value method here is calculated as a perpetuity - it will go on forever, decreasing in value only slightly by 2% per year, reflecting that the growth rate does not keep pace with the required rate of return. {Mathematically, saying that a perpetuity = FCF / discount rate is merely the mathematical simplification of FCF1 / (1-r)^1 + FCF2 / (1-r)^2 + ... FCF [infinity] / (1-r)^[infinity] ; you can test this yourself by calculating the value of cashflows over 1,000 years compared with the perpetuity formula and see that it is the same thing}

$100 / .02 = $5,000. This is the value of the asset to you today - it is not the value of the asset in 364 days. It is what you should pay, today, for an income stream of those properties.

Now say that you would earn $50 in 12 months, and then get the same terminal value per above, starting in 366 days. Your valuation of the total FCF would be $50 / (1-.04) + $5,000 / (1-.04). Note that the Terminal value is discounted back to today's dollars using the same discount rate as the cash expected for the end of this year - because the perpetuity calculation brings those values into 'current value' as of 366 days from now.

The same is for your example - year 4 cashflows and year 5 terminal value are discounted back using the same rate, because year 4 FCF represents the cash received at the end of year 4, and year 5 terminal value represents the value of cash as of the 1st day of year 5.

Answer 3

I managed to derive the formula for the terminal value in the special case of g = 0.

In the special case of g = 0, the terminal value in the question above is the sum of this geometric series:

terminal value = [FCF_5 / (1 + r)^5] + [FCF_5 / (1 + r)^6] + [FCF_5 / (1 + r)^7] + ...

Or equivalently:

terminal value = (FCF_5 / (1 + r)^5) (1 + [1 / (1 + r)^1] + [1 / (1 + r)^2] + ...)

Let s = (1 + [1 / (1 + r)^1] + [1 / (1 + r)^2] + ...), so

terminal value = (FCF_5 / (1 + r)^5) (s)

To calculate the sum of the geometric series s, use the formula for the geometric series as the number of terms approaches infinity:

s = α / (1 - β), for |β| < 1

Substituting α = 1 and common ratio β = 1 / (1 + r) into the geometric series formula and rearranging, we get:

s = (1 + r) / r

Now, substitute s and rearrange to get the formula for the terminal value:

terminal value = (FCF_5 / (1 + r)^5) (s)

terminal value = (FCF_5 / (1 + r)^5) ([1 + r] / r)

terminal value = FCF_5 / [(1 + r)^4 (r)]

---

I also managed to derive the formula for the terminal value as shown in the question (where g can be ≠ 0).

The terminal value in the question above is the sum of this geometric series, with free cash flow growing by a constant percentage g every year:

terminal value = [FCF_5 / (1 + r)^5] + [(FCF_5)(1 + g)^1 / (1 + r)^6] + [(FCF_5)(1 + g)^2 / (1 + r)^7] + ...

Or equivalently:

terminal value = [FCF_5 / (1 + r)^5] (1 + [(1 + g) / (1 + r)]^1 + [(1 + g) / (1 + r)]^2 + ...)

Let s = (1 + [(1 + g) / (1 + r)]^1 + [(1 + g) / (1 + r)]^2 + ...), so

terminal value = (FCF_5 / (1 + r)^5) (s)

To calculate the sum of the geometric series s, use the formula for the geometric series as the number of terms approaches infinity:

s = α / (1 - β), for |β| < 1

Substituting α = 1 and common ratio β = (1 + g) / (1 + r) into the geometric series formula and rearranging, we get:

s = (1 + r) / (r - g)

Now, substitute s and rearrange to get the formula for the terminal value:

terminal value = (FCF_5 / (1 + r)^5) (s)

terminal value = (FCF_5 / (1 + r)^5) ([1 + r] / [r - g])

terminal value = FCF_5 / [(1 + r)^4 (r - g)]

---

Todo:

• Find an intuitive explanation of the formula.