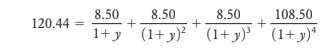Yield to maturity (YTM) for a bond - definition

Question

In "Principles of corporate finance" (Brealey, Myers, Allen) the YTM corresponding to a currently priced bond is the "y" unknown from the formula:

(present value of a 8.5% coupon bond - sold at a premium to face value of 100)

This takes into account the actual "equivalent" discount rate that justifies the present value (PV) of the security.

Looking in Investopedia for yield to maturity article I see the following addition though "YTM assumes that all coupon payments are reinvested at a yield equal to the YTM and that the bond is held to maturity"

I wonder what is the proper definition though. In the cash flow model if you want to take into account reinvesting coupons it becomes interesting as you need to solve for "y" considering that at every cash flow point you reinvest with the same yield. (curios also with the math that involves investing coupons).

Answer 1

The definitions are both correct. The reason for the assumption is to make the bond equivalent in value to just investing the market price at the YTM compounded continuously. So the coupons received are cancelled out by investing that cash in something else with the same yield.

If you don't reinvest the coupons, you'll have a different ending value - you'll just have the face value of the bond plus the coupons. So the initial coupons just sit as cash until the bond matures, when in reality, investors will take the coupons cash and reinvest it somewhere.

In other words, a 5-year bond with a YTM of 5% that costs $97 is equivalent to (cash flow wise) putting $97 in a 2% savings account for 5 years. Reinvesting the coupons cancels out the cash flows, and you'll have the same enging value in cash at the maturity of the bond.

Answer 2

It is a common fallacy to state the reinvestment assumption. Some papers trying to address this problem.

• The controversial reinvestment assumption in IRR and NPV estimates: New evidence against reinvestment assumption; Arjunan and Kannapiran
• The IRR, NPV and the Fallacy of the Reinvestment Rate Assumptions; Lohmann
• The Internal Rate of Return and the Reinvestment Fallacy; Keane
• Yield-to-Maturity and the Reinvestment of Coupon Payments; Shawn M. Forbes, John J. Hatem, and Chris Paul
• The Reinvestment Rate Assumption Fallacy for IRR and NPV: A Pedagogical Note; Magni, Carlo Alberto and Martin, John D.

All NPV of a bond (and internal rate of retrn: IRR) does is to discount cashflows. It is just trying to measure the return offered by a project taking into account the timings of cash flows. The discount rate that matches the quoted NPV of a bond is the YTM. As soon as scenarios on how the interim cash flows might be used are included in the calculation of NPV (YTM or IRR), you would be calculating the NPV (or IRR) of a different set of cashflows. Hence, there is no separate accounting for reinvested cashfows or other stuff.

The confusion comes from the observation that the YTM (IRR) is not equal to the total profit expressed in percent. However, a 5% bond also just pays a 5% coupon rate every year. If YTM is also 5% it is priced at par. Yet, if your bond has a maturity date in 5 years, you do not get 100*(1,05)^5 ~ 127,63 but simply 5*5+100 = 125 if interest is paid annually.

YTM just expresses the bond coupon (instead of the 5%) in a comparable manner, taking the price of the bond into account.